Pull That Up, Jamie

Einstein’s Great Insight: General Relativity

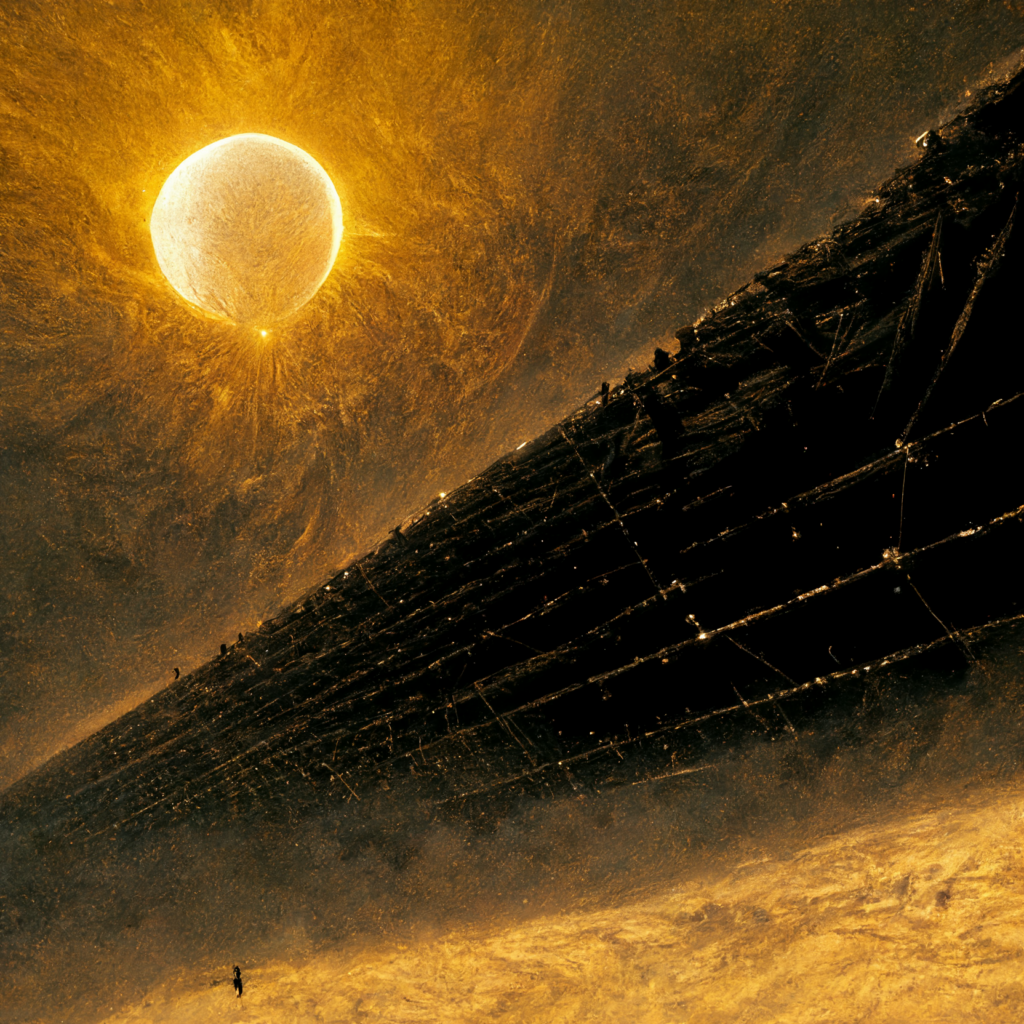
The “curvature” ship mnemonically represents that curvature can be decomposed into a sort of sum of what are called the Weyl tensor, Traceless Ricci, and Ricci Scalar considered as elements of “adjoint valued” (tensor product of adjoint bundle with form bundle) 2-forms. The ship is being placed in a bottle labelled under the symmetric 2 forms on the cotangent bundle of X.

General Relativity vs Gauge Theory Incompatibility

Distinguishing this from the previous is a different choice of what is meant by curvature, the same ship is placed in the set of d-1 degree adjoint valued forms.

Geometric Unity for General Relativity & Gauge Theory

The algebraic incompatibility between what is done in these two cases to curvature is to create a “Shiab Projection” operation to “preserve the properties of the masts” such that they survive when made into a connection/d-1 form.

Curvature in Gauge Theory: “Penrose-like steps”

Gauge theories are theories of differential calculus. In the usual formulation of ordinary calculus we say that the derivative of a single variable function f(x) gives the instantaneous slope of a function as ‘Rise/Run’. Gauge theory, by contrast, says “Not so fast. When you say ‘Rise over Run’ you didn’t say from what reference level the ‘Rise’ is to be measured: it should be  ‘(Rise above Reference)/Run’. This opens the door to the idea that the reference level is actually a line that is at the very heart of the derivative but has been omitted and set equal to the default horizontal line rather than allowing the user to choose the best reference level from the description of the problem. When calculus moves from one to two variables, a function f(x,y) has its derivatives as “(Rises above a Reference *Plane*)/Runs” rather than a reference line. Here a system of such reference planes is shown from a problem in Gauge Theoretic Economics.

Gauge Theory as Calculus Done Right (Pt. 1)

Here is shown a red curve/function, possibly representing the inflation of a currency as time progresses to the right along with a blue function representing the notion of “constant wage.” The concept at play is that the definition of constant depends on the faded horizontal ticks in the background space, and in the example of a wage, it makes conceptual sense to set such a notion of constant against the inflation curve rather than the axis. This represents one spirit of what is done in the “calculus of gauges,” and in reality we tend not to see god-given coordinate grids sprawling over space thus encouraging us to adopt a different language of geometry/calculus.

Gauge Theory Sections Replace Functions (Pt. 2)

The familiar trig functions have a property of periodicity to them – they repeat their values after enough change to progress their inputs rightwards. Because of this, it is enough to study a finite part of them between repeating periods, and plot this on a cylinder. Now the essential new structure of gauge theory over calculus is that what we consider to be a graph is weakened, and is just some curve that horizontally traverses through a space projectible to the inputs of the new function. This is realized here as both the cylinder and mobius band, both projectible to the black horizontal “base.” What structure we retain from ordinary calculus is there being a value of a graph corresponding to each point on the black base and the possible values can be thought of as follows: The cylinder can be thought of as starting with only the black circle, and then attaching the midpoint of a short line segment to each point of the black circle. It follows that functions are just choices of one point for each of these vertical “fibers.” We try to construct a new surface where the fibers tilt slowly over the base enough as to flip their orientation as they come around. The analogous sine and cosine graphs inherited from the cylinder fail to be continuous, so they must also be analogously modified. The theme that this example teaches us about geometry further is that the possible continuous graphs of functions are characterized by the kind of space they are graphed in and vice versa.

Gauge Group Acting on Principal U(1) Bundle

Combining the qualities of the last two examples, defining a new “horizontal” and considering new types of spaces with new graphs we have the transformation to a horizontal choice on a collection of fibers over the horizontal circle. The reason why the new fibers chosen are now closed loops is technical but simple – you can transform each fiber onto itself via rotation in such a way the line segment cannot, naming this loop with the ability to transform U(1). The transformation of our red level and associated blue parallel levels spaced evenly around the shown green fibers demonstrates the flexibility of a gauge transformation, it appears a little differently as you traverse the horizontals.

Gauge Transformation Acts on Section and Connection

Amending the language of previous examples, the horizontal black circle will be referred to as the base, the rotatable green loops along with their unmarked infinite family will be called fibers associated to points on the base. The ensemble of the fibers and base woven together into a larger continuous object pictured here as the donut is a fiber bundle. As we have seen, each fiber transforms individually, and similarly to its adjacent neighbors – but what we lack is an ability to directly relate neighboring fibers in the same way we need to take differences in calculus between the values of a function over two different inputs and then construct tangents or derivatives. The choice of red horizontal now to be called a section (across the fibers) is actually enough to do this horizontal traversal, since with all of its parallels produced by gauge-transforming it around the fibers gives an association of points everywhere in each fiber onto the other fibers of your choice. Now for the namesake of the animation, the only difference between the red section and the whole connection is that the connection is only recoverable from the section, but covering all points of each fiber – represented by the blue ticks on the fibers that are shown.

Water Wiggler = U(1) Principal Bundle over S¹

U(1) G-action on Water Wiggle Principal Bundle

A final piece of terminology for bundles – U(1) was used to describe the circle fibers with their transformations that map to themselves, S1 refers to a barer structure of the circle – being intuitively connected and finite in extent with no boundary. Now we can finally say like a mathematician, we have been studying a U(1) Principal Bundle over S1. The animations of the water wiggler, as is and with fibers/sections highlighted show a slight amount more rigidity than the previous abstract U(1) bundle/donut – the plastic composing it would tear or stretch if the gauge transformation did not respect the added stiffness of the material, seen in the lines drawn in the latter as it rolls on itself.

Square Becomes 2D Model of Spacetime

The donut/torus can be constructed via patching the boundary of a square in a particularly configured way – arrows on what is called the fundamental polygon would tell you what orientation to connect such that you get a donut, sphere, projective plane, or klein bottle.

Metrics As The Difference Between a Donut and Mug

When introducing people to the term “topology,” the example given is that topology is like “rubber sheet geometry” – then to relate a coffee mug to a donut. What mathematics really does is to stratify all of the parts of traditional geometry into their essential parts, and here we see that from the barest parts, the way a space is put together by rubber-like circular patches and the addition of what we call a metric. The water wiggler introduced one aspect to this, the rigidity we enforced on the fiber/section curves and in another way the lack of it when deforming the whole shape. At the intersection between these two kinds of rigidity is what we do to transform the coffee mug into the torus. The conceptual coordinate lines (really fiber/section) show what is meant – the squares formed by coordinate lines may bulge out like those on a globe (lat/long), the angles between them may deform, and lengths may stretch nonuniformly. Similarly to how vectors can be thought of as lists of components in each given direction, such numerical information organized by what physical directions it’s associated with we call a metric. The coordinate data for a metric as hinted before may only exist for patches (notice the longitude lines on a globe converging at a point, latitude becomes null). This metric information is naturally associated with a field of frames as in “Penrose-Like Steps.”

2D Space-Time v Space-Space/Time-Time Metrics

To get a firmer grip on what metrics do, we look at the possible metrics on only one point of the donut. To encompass what was demanded of metrics in the previous example with lengths and angles we state that a metric associates pairs of vectors (maybe in a frame) to a value indicating some aspect of the shape of this frame, and since we are only qualifying the shape of this pairwise frame we do not care if we choose vector 2 and then 1 or vice versa. Measuring the vectors tangent to a point on the torus, they are built out of some amount of each vector in the frame – so we have four numbers (two from each, two numbers to describe the direction and length of each vector) and correspondingly get that a metric has four numbers telling you how to take pythagoras’ formula at the point on the donut. The condition that we do not care about the order of the vectors taken in by the metric sets two of these four numbers equal, leaving 3 distinct possible numerical choices and letting us to visualize the collection of these metrics as points in 3d space. The length of a single vector can be extracted by plugging it in twice to the metric to get the pythagorean sum of squares. What is shown in this animation are the different kinds of possible 2d metrics, separated by a red/blue double cone defined by the equation xy=z^2 from the three choices of components of the metric. If your choice of metric lies on this cone, it will always say the length of a vector is zero regardless of the vector. Test it by hand! Inside one cone are the metrics that take strictly positive values, negative in the other, and the green space taking mixed values.

Picturing Different Space-Time Metrics in 2D

The actual pythagorean formula for a vector (a,b) is given by a^2+b^2, so you can deduce the components of the pythagorean metric are x=1, y=1, z=0 – but all of the strictly positive metrics are “equivalent” in a mathematical sense. When we do Einstein’s Relativity, we want to distinguish the space and time dimensions/components of vectors since we otherwise regard time as another geometric dimension – this is done by assigning vectors in the time direction with negative lengths and space-like vectors with positive lengths.

To reiterate the purpose of the three components of the 2D metric, a frame corresponds to a metric by picking the values of the metric as the values we want for the length of vector 1, vector 2, and their combination (related to their angle) as the z component. One of these vectors can be associated with the time direction on the donut and one with space, and we can do relativity on the donut! We have done a lot of work to understand one metric value at a point, but now it is easy to pick a continuous collection of metrics for the whole donut – we just place the donut in the metric space as shown here.

2-D S-T Metric (at a Point x) = 2 Rulers + 1 Protractor

5D Observerse Replaces SpaceTime (Shown For 2D)

Congratulations you have picked up the weight of our mathematical language and can run now, shown for some individual points on the donut are choices of spacetime metrics in the cone space along with their corresponding ruler/protractor.